Compositions of Functions
Learning Outcomes
- Combine functions using algebraic operations.
- Create a new function by composition of functions.
- Evaluate composite functions.
- Find the domain of a composite function.
- Decompose a composite function into its component functions.
 Figure 1
Figure 1Compositions of Functions
Function composition is only one way to combine existing functions. Another way is to carry out the usual algebraic operations on functions, such as addition, subtraction, multiplication and division. We do this by performing the operations with the function outputs, defining the result as the output of our new function.
Suppose we need to add two columns of numbers that represent a husband and wife’s separate annual incomes over a period of years, with the result being their total household income. We want to do this for every year, adding only that year’s incomes and then collecting all the data in a new column. If is the wife’s income and is the husband’s income in year , and we want to represent the total income, then we can define a new function.
If this holds true for every year, then we can focus on the relation between the functions without reference to a year and write
Just as for this sum of two functions, we can define difference, product, and ratio functions for any pair of functions that have the same kinds of inputs (not necessarily numbers) and also the same kinds of outputs (which do have to be numbers so that the usual operations of algebra can apply to them, and which also must have the same units or no units when we add and subtract). In this way, we can think of adding, subtracting, multiplying, and dividing functions.
For two functions and with real number outputs, we define new functions , and by the relations
Example: Performing Algebraic Operations on Functions
Find and simplify the functions and , given and . Give the domain of your result. Are they the same function?Answer:
Begin by writing the general form, and then substitute the given functions.
Note: For , the condition is necessary because when , , which makes the quotient function undefined (dividing by zero).
Try It
Find and simplify the functions and .Are they the same function?
Answer:
No, the functions are not the same.try it
Let's explore what happens to the graphs of functions that are combined using algebraic operations. Use an online graphing tool to graph the following functions:Create a New Function Using a Composition
We read the left-hand side as composed with at and the right-hand side as of of The two sides of the equation have the same mathematical meaning and are equal. The open circle symbol is called the composition operator. We use this operator mainly when we wish to emphasize the relationship between the functions themselves without referring to any particular input value. Composition is a binary operation that takes two functions and forms a new function, much as addition or multiplication takes two numbers and gives a new number. However, it is important not to confuse function composition with multiplication because, as we learned above, in most cases . It is also important to understand the order of operations in evaluating a composite function. We follow the usual convention with parentheses by starting with the innermost parentheses first, and then working to the outside. In the equation above, the function takes the input first and yields an output . Then the function takes as an input and yields an output .
 Figure 2
Figure 2\begin{align}f\left(g\left(x\right)\right)&=f\left(x+2\right) \\[2mm] &={\left(x+2\right)}^{2} \\[2mm] &={x}^{2}+4x+4\hfill \end{align}
but
\begin{align}g\left(f\left(x\right)\right)&=g\left({x}^{2}\right) \\[2mm] \text{ }&={x}^{2}+2\hfill \end{align}
These expressions are not equal for all values of , so the two functions are not equal. It is irrelevant that the expressions happen to be equal for the single input value . Note that the range of the inside function (the first function to be evaluated) needs to be within the domain of the outside function. Less formally, the composition has to make sense in terms of inputs and outputs. Additionally, in applied settings, function composition usually only makes sense in one specific order.A General Note: Composition of Functions
When the output of one function is used as the input of another, we call the entire operation a composition of functions. For any input and functions and , this action defines a composite function, which we write as such thatThe domain of the composite function is all such that is in the domain of and is in the domain of . It is important to realize that the product of functions is not the same as the function composition , because, in general, .
Example: Define a Composition of Functions
Using the functions provided, find and .Answer: To determine , we substitute into . Now we can determine by substituting into .
try it
Now we will explore the graph of a composition of functions. Use an online graphing tool to graph the following functions:Now zoom in (use the plus sign in the upper right-hand corner of the graph) on the point , notice that both graphs pass through this point. Continue this pattern for a few more iterations: define a new function that contains another composition of with itself. For example: , and so on. Continue to use the zoom as you iterate. The point is called a fixed point of the function . Fixed points are used in mathematical applications such as the page rank algorithm that Google uses to generate internet search results. Read more about fixed points here, and page rank here.
Example: Interpreting Composite Functions
The function gives the number of calories burned completing sit-ups, and gives the number of sit-ups a person can complete in minutes. Interpret .Answer: The inside expression in the composition is . Because the input to the s-function is time, represents 3 minutes, and is the number of sit-ups completed in 3 minutes. Using as the input to the function gives us the number of calories burned during the number of sit-ups that can be completed in 3 minutes, or simply the number of calories burned in 3 minutes (by doing sit-ups).
Example: Investigating the Order of Function Composition
Suppose gives miles that can be driven in hours and gives the gallons of gas used in driving miles. Which of these expressions is meaningful: orAnswer: The function is a function whose output is the number of miles driven corresponding to the number of hours driven. The function is a function whose output is the number of gallons used corresponding to the number of miles driven. This means: The expression takes miles as the input and a number of gallons as the output. The function requires a number of hours as the input. Trying to input a number of gallons does not make sense. The expression is meaningless. The expression takes hours as input and a number of miles driven as the output. The function requires a number of miles as the input. Using (miles driven) as an input value for , where gallons of gas depends on miles driven, does make sense. The expression makes sense, and will yield the number of gallons of gas used, , driving a certain number of miles, , in hours.
Q & A
Are there any situations where and would both be meaningful or useful expressions? Yes. For many pure mathematical functions, both compositions make sense, even though they usually produce different new functions. In real-world problems, functions whose inputs and outputs have the same units also may give compositions that are meaningful in either order.Try It
The gravitational force on a planet a distance r from the sun is given by the function . The acceleration of a planet subjected to any force is given by the function . Form a meaningful composition of these two functions, and explain what it means.Answer: A gravitational force is still a force, so makes sense as the acceleration of a planet at a distance r from the Sun (due to gravity), but does not make sense.
Evaluate a Composition of Functions
Once we compose a new function from two existing functions, we need to be able to evaluate it for any input in its domain. We will do this with specific numerical inputs for functions expressed as tables, graphs, and formulas and with variables as inputs to functions expressed as formulas. In each case we evaluate the inner function using the starting input and then use the inner function’s output as the input for the outer function.Evaluating Composite Functions Using Tables
When working with functions given as tables, we read input and output values from the table entries and always work from the inside to the outside. We evaluate the inside function first and then use the output of the inside function as the input to the outside function.Example: Using a Table to Evaluate a Composite Function
Using the table below, evaluate and .| 1 | 6 | 3 |
| 2 | 8 | 5 |
| 3 | 3 | 2 |
| 4 | 1 | 7 |
Answer: To evaluate , we start from the inside with the input value 3. We then evaluate the inside expression using the table that defines the function . We can then use that result as the input to the function , so is replaced by 2 and we get . Then, using the table that defines the function , we find that .
\begin{align}&g\left(3\right)=2 \\[1.5mm]& f\left(g\left(3\right)\right)=f\left(2\right)=8\end{align}
To evaluate , we first evaluate the inside expression using the first table: . Then, using the table for , we can evaluateThe table below shows the composite functions and as tables.
| 3 | 2 | 8 | 3 | 2 |
Try It
Using the table below, evaluate and .| 1 | 6 | 3 |
| 2 | 8 | 5 |
| 3 | 3 | 2 |
| 4 | 1 | 7 |
Answer:
Evaluating Composite Functions Using Graphs
When we are given individual functions as graphs, the procedure for evaluating composite functions is similar to the process we use for evaluating tables. We read the input and output values, but this time, from the and axes of the graphs.How To: Given a composite function and graphs of its individual functions, evaluate it using the information provided by the graphs.
- Locate the given input to the inner function on the axis of its graph.
- Read off the output of the inner function from the axis of its graph.
- Locate the inner function output on the axis of the graph of the outer function.
- Read the output of the outer function from the axis of its graph. This is the output of the composite function.
Example: Using a Graph to Evaluate a Composite Function
Using the graphs below, evaluate .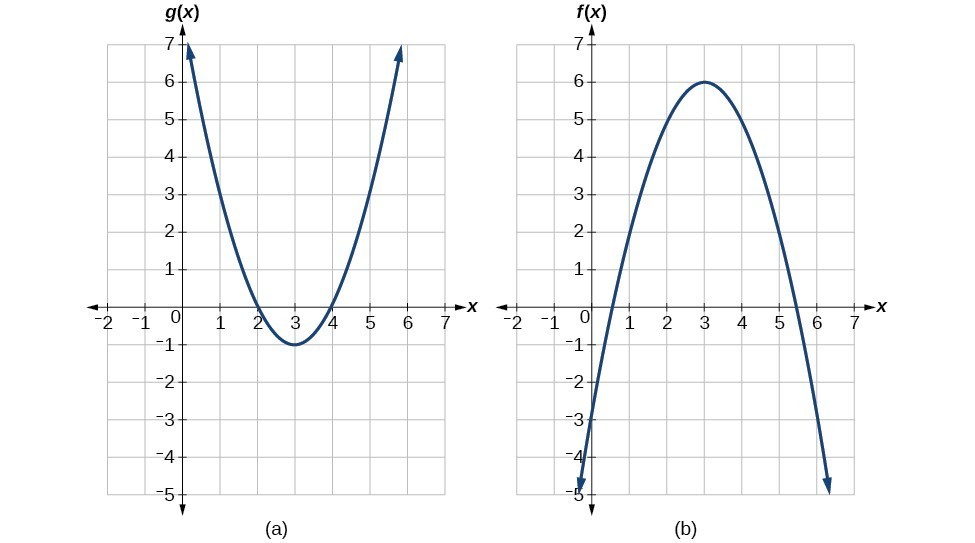
Answer:
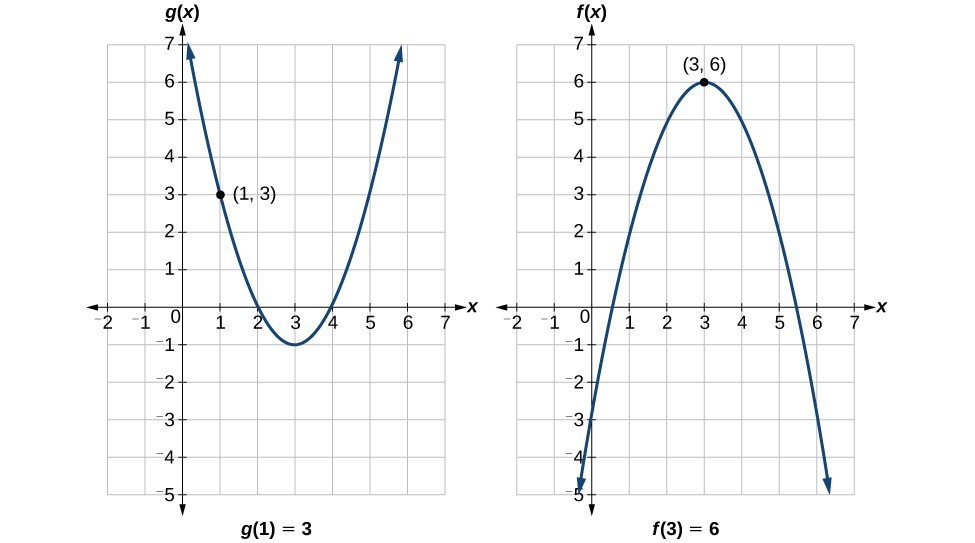 To evaluate , we start with the inside evaluation.
We evaluate using the graph of , finding the input of 1 on the axis and finding the output value of the graph at that input. Here, . We use this value as the input to the function .
To evaluate , we start with the inside evaluation.
We evaluate using the graph of , finding the input of 1 on the axis and finding the output value of the graph at that input. Here, . We use this value as the input to the function .
We can then evaluate the composite function by looking to the graph of , finding the input of 3 on the axis and reading the output value of the graph at this input. Here, , so .
Analysis of the Solution
The figure shows how we can mark the graphs with arrows to trace the path from the input value to the output value.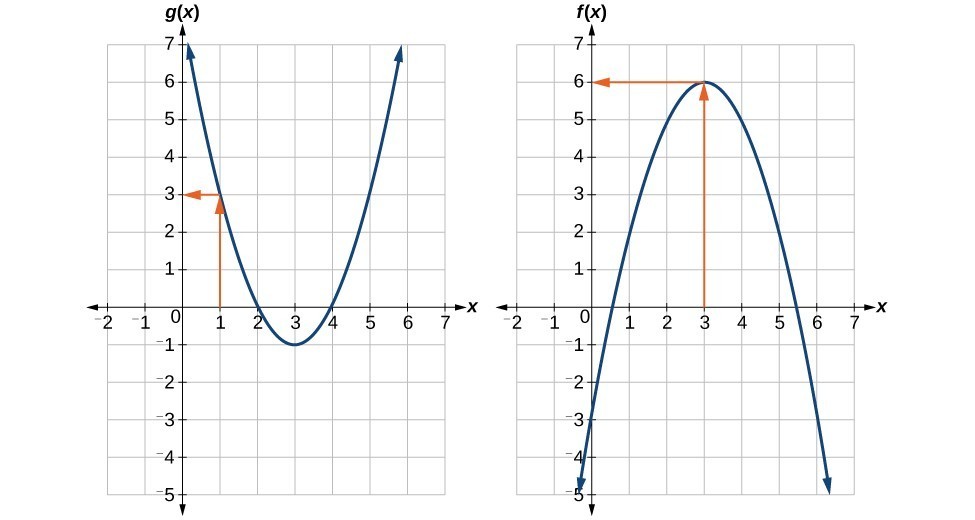
Try It
Using the graphs below, evaluate .
Answer:
Evaluating Composite Functions Using Formulas
When evaluating a composite function where we have either created or been given formulas, the rule of working from the inside out remains the same. The input value to the outer function will be the output of the inner function, which may be a numerical value, a variable name, or a more complicated expression. While we can compose the functions for each individual input value, it is sometimes helpful to find a single formula that will calculate the result of a composition . To do this, we will extend our idea of function evaluation. Recall that, when we evaluate a function like , we substitute the value inside the parentheses into the formula wherever we see the input variable.How To: Given a formula for a composite function, evaluate the function.
- Evaluate the inside function using the input value or variable provided.
- Use the resulting output as the input to the outside function.
Example: Evaluating a Composition of Functions Expressed as Formulas with a Numerical Input
Given and , evaluate .Answer: Because the inside expression is , we start by evaluating at 1.
\begin{align}h\left(1\right)&=3\left(1\right)+2\\[2mm] h\left(1\right)&=5\end{align}
Then , so we evaluate at an input of 5.\begin{align}f\left(h\left(1\right)\right)&=f\left(5\right)\\[2mm] f\left(h\left(1\right)\right)&={5}^{2}-5\\[2mm] f\left(h\left(1\right)\right)&=20\end{align}
Analysis of the Solution
It makes no difference what the input variables and were called in this problem because we evaluated for specific numerical values.Try It
Given and , evaluate A) B)Answer: A. 8; B. 20 You can check your work with an online graphing tool. Enter the functions above into a graphing calculator as they are defined. In the next line enter . You should see in the bottom right corner.
Domain of a Composition
As we discussed previously, the domain of a composite function such as is dependent on the domain of and the domain of . It is important to know when we can apply a composite function and when we cannot, that is, to know the domain of a function such as . Let us assume we know the domains of the functions and separately. If we write the composite function for an input as , we can see right away that must be a member of the domain of in order for the expression to be meaningful, because otherwise we cannot complete the inner function evaluation. However, we also see that must be a member of the domain of , otherwise the second function evaluation in cannot be completed, and the expression is still undefined. Thus the domain of consists of only those inputs in the domain of that produce outputs from belonging to the domain of . Note that the domain of composed with is the set of all such that is in the domain of and is in the domain of .A General Note: Domain of a Composite Function
The domain of a composite function is the set of those inputs in the domain of for which is in the domain of .How To: Given a function composition , determine its domain.
- Find the domain of g.
- Find the domain of f.
- Find those inputs, , in the domain of for which is in the domain of . That is, exclude those inputs, , from the domain of for which is not in the domain of . The resulting set is the domain of .
Example: Finding the Domain of a Composite Function
Find the domain of
Answer: The domain of consists of all real numbers except , since that input value would cause us to divide by 0. Likewise, the domain of consists of all real numbers except 1. So we need to exclude from the domain of that value of for which .
\begin{align}&\dfrac{4}{3x - 2}=1\hspace{5mm}&&\text{Set}\hspace{2mm}g(x)\hspace{2mm}\text{equal to 1} \\[2mm]& 4=3x - 2 &&\text{Multiply by}\hspace{2mm} 3x-2\\[2mm]& 6=3x&&\text{Add 2 to both sides}\\[2mm]& x=2&&\text{Divide by 3} \end{align}
So the domain of is the set of all real numbers except and . This means thatWe can write this in interval notation as
Example: Finding the Domain of a Composite Function Involving Radicals
Find the domain of
Answer: Because we cannot take the square root of a negative number, the domain of is . Now we check the domain of the composite function
For , we need , since the radicand of a square root must be positive. Since square roots are positive, , or , which gives a domain of .
Analysis of the Solution
This example shows that knowledge of the range of functions (specifically the inner function) can also be helpful in finding the domain of a composite function. It also shows that the domain of can contain values that are not in the domain of , though they must be in the domain of . You cannot rely on an algorithm to find the domain of a composite function. Rather, you will need to first ask yourself "what is the domain of the inner function", and determine whether this set will comply with the domain restrictions of the outer function. In this case, the set ensures a non-negative output for the inner function, which will in turn ensure a positive input for the composite function.Try It
Find the domain of
Answer:
[ohm_question hide_question_numbers=1]1603[/ohm_question] [ohm_question hide_question_numbers=1]1604[/ohm_question]try it
We can use graphs to visualize the domain that results from a composition of two functions. Graph the two functions below with an online graphing tool.Decompose a Composite Function
In some cases, it is necessary to decompose a complicated function. In other words, we can write it as a composition of two simpler functions. There is almost always more than one way to decompose a composite function, so we may choose the decomposition that appears to be most obvious.Example: Decomposing a Function
Write as the composition of two functions.Answer: We are looking for two functions, and , so . To do this, we look for a function inside a function in the formula for . As one possibility, we might notice that the expression is the inside of the square root. We could then decompose the function as
We can check our answer by recomposing the functions.
Analysis of the Solution
For every composition there are infinitely many possible function pairs that will work. In this case, another function pair where is andTry It
Write as the composition of two functions.Answer: Possible answer:
Key Equation
| Composite function |
Key Concepts
- We can perform algebraic operations on functions.
- When functions are combined, the output of the first (inner) function becomes the input of the second (outer) function.
- The function produced by combining two functions is a composite function.
- The order of function composition must be considered when interpreting the meaning of composite functions.
- A composite function can be evaluated by evaluating the inner function using the given input value and then evaluating the outer function taking as its input the output of the inner function.
- A composite function can be evaluated from a table.
- A composite function can be evaluated from a graph.
- A composite function can be evaluated from a formula.
- The domain of a composite function consists of those inputs in the domain of the inner function that correspond to outputs of the inner function that are in the domain of the outer function.
- Just as functions can be combined to form a composite function, composite functions can be decomposed into simpler functions.
- Functions can often be decomposed in more than one way.
Glossary
- composite function
- the new function formed by function composition, when the output of one function is used as the input of another
