Coordinate Plane and Graphing Equations

Learning Objectives

Create a table of ordered pairs from a two-variable linear equation
Graph a two-variable linear equation using a table of ordered pairs
Determine whether an ordered pair is a solution of an equation
Recognize when an ordered pair is a y-intercept or an x-intercept
Graph a linear equation using x- and y-intercepts
Graphing other equations using a table or ordered pairs

The coordinate plane was developed centuries ago and refined by the French mathematician René Descartes. In his honor, the system is sometimes called the Cartesian coordinate system. The coordinate plane can be used to plot points and graph lines. This system allows us to describe algebraic relationships in a visual sense, and also helps us create and interpret algebraic concepts. You have likely used a coordinate plane before. The coordinate plane consists of a horizontal axis and a vertical axis, number lines that intersect at right angles. 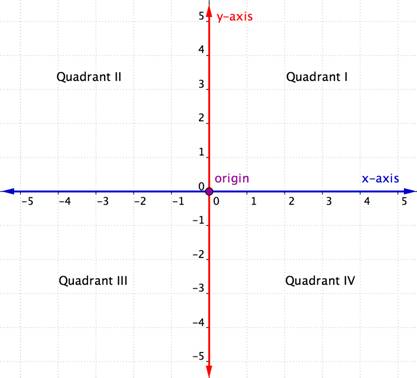(They are perpendicular to each other.) A graph with an x-axis running horizontally and a y-axis running vertically. The location where these axes cross is labeled the origin, and is the point zero, zero. The axes also divide the graph into four equal quadrants. The top right area is quadrant one. The top left area is quadrant two. The bottom left area is quadrant three. The bottom right area is quadrant four.

A graph with an x-axis running horizontally and a y-axis running vertically. The location where these axes cross is labeled the origin, and is the point zero, zero. The axes also divide the graph into four equal quadrants. The top right area is quadrant one. The top left area is quadrant two. The bottom left area is quadrant three. The bottom right area is quadrant four.

The horizontal axis in the coordinate plane is called the x-axis. The vertical axis is called the y-axis. The point at which the two axes intersect is called the origin. The origin is at 0 on the x-axis and 0 on the y-axis. Locations on the coordinate plane are described as ordered pairs. An ordered pair tells you the location of a point by relating the point’s location along the x-axis (the first value of the ordered pair) and along the y-axis (the second value of the ordered pair). In an ordered pair, such as (x, y), the first value is called the x-coordinate and the second value is the y-coordinate. Note that the x-coordinate is listed before the y-coordinate. Since the origin has an x-coordinate of 0 and a y-coordinate of 0, its ordered pair is written (0, 0). Consider the point below. Grid with x-axis and y-axis. A blue dotted line extends from the origin, which is the point (0,0) along the horizontal x-axis to 4. A red dotted line goes up vertically from 4 on the x-axis to 3 on the y-axis. That point is labeled (4, 3).

Grid with x-axis and y-axis. A blue dotted line extends from the origin, which is the point (0,0) along the horizontal x-axis to 4. A red dotted line goes up vertically from 4 on the x-axis to 3 on the y-axis. That point is labeled (4, 3).

To identify the location of this point, start at the origin (0, 0) and move right along the x-axis until you are under the point. Look at the label on the x-axis. The 4 indicates that, from the origin, you have traveled four units to the right along the x-axis. This is the x-coordinate, the first number in the ordered pair. From 4 on the x-axis move up to the point and notice the number with which it aligns on the y-axis. The 3 indicates that, after leaving the x-axis, you traveled 3 units up in the vertical direction, the direction of the y-axis. This number is the y-coordinate, the second number in the ordered pair. With an x-coordinate of 4 and a y-coordinate of 3, you have the ordered pair (4, 3).

Example

Describe the point shown as an ordered pair. A point that is 2 spaces above the x-axis and 5 spaces to the right of the y-axis.

Answer: Begin at the origin and move along the x-axis. This is the x-coordinate and is written first in the ordered pair.

(5, y)

Move from 5 up to the ordered pair and read the number on the y-axis. This is the y-coordinate and is written second in the ordered pair.

(5, 2)

Answer

The point shown as an ordered pair is (5, 2).

Example

Plot the point 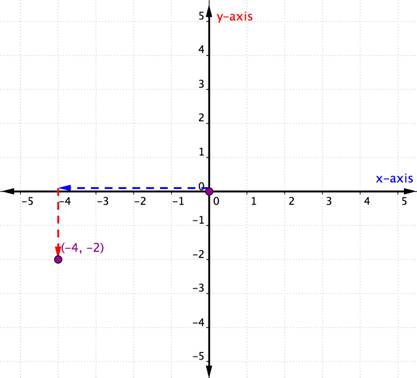[latex](−4,−2)[/latex]. Graph with blue arrow pointing from origin to four units to the left. A red arrow points down 2 spaces to the point negative 4, negative 2.

Graph with blue arrow pointing from origin to four units to the left. A red arrow points down 2 spaces to the point negative 4, negative 2.

The x-coordinate is [latex]−4[/latex] because it comes first in the ordered pair. Start at the origin and move 4 units in a negative direction (left) along the x-axis. The y-coordinate is [latex]−2[/latex] because it comes second in the ordered pair. Now move 2 units in a negative direction (down). If you look over to the y-axis, you should be lined up with [latex]−2[/latex] on that axis.

Answer: Draw a point at this location and label the point [latex](−4,−2)[/latex].

https://youtu.be/p_MESleS3mw Graphing ordered pairs is only the beginning of the story. Once you know how to place points on a grid, you can use them to make sense of all kinds of mathematical relationships.

You can use a coordinate plane to plot points and to map various relationships, such as the relationship between an object’s distance and the elapsed time. Many mathematical relationships are linear relationships. Let’s look at what a linear relationship is.

Plotting points to graph linear relationships

A linear relationship is a relationship between variables such that when plotted on a coordinate plane, the points lie on a line. Let’s start by looking at a series of points in Quadrant I on the coordinate plane. These series of points can also be represented in a table. In the table below, the x- and y-coordinates of each ordered pair on the graph is recorded.

x-coordinate	y-coordinate
0	0
1	2
2	4
3	6
4	8

Notice that each y-coordinate is twice the corresponding x-value. All of these x- and y-values follow the same pattern, and, when placed on a coordinate plane, they all line up. Graph with the point (0,0); the point (1,2); the point (2,4); the point (3,6); and the point (4,8).

Graph with the point (0,0); the point (1,2); the point (2,4); the point (3,6); and the point (4,8).

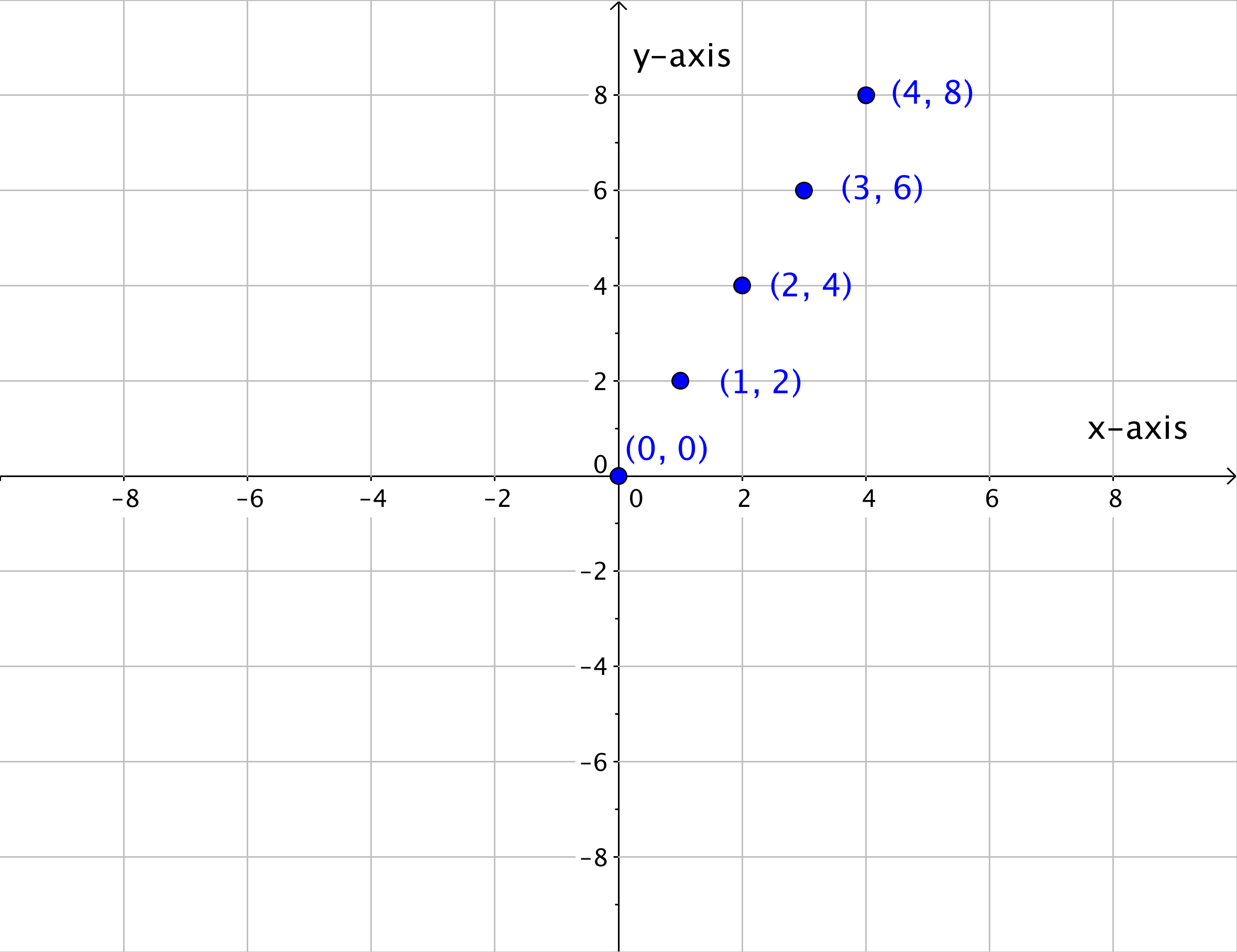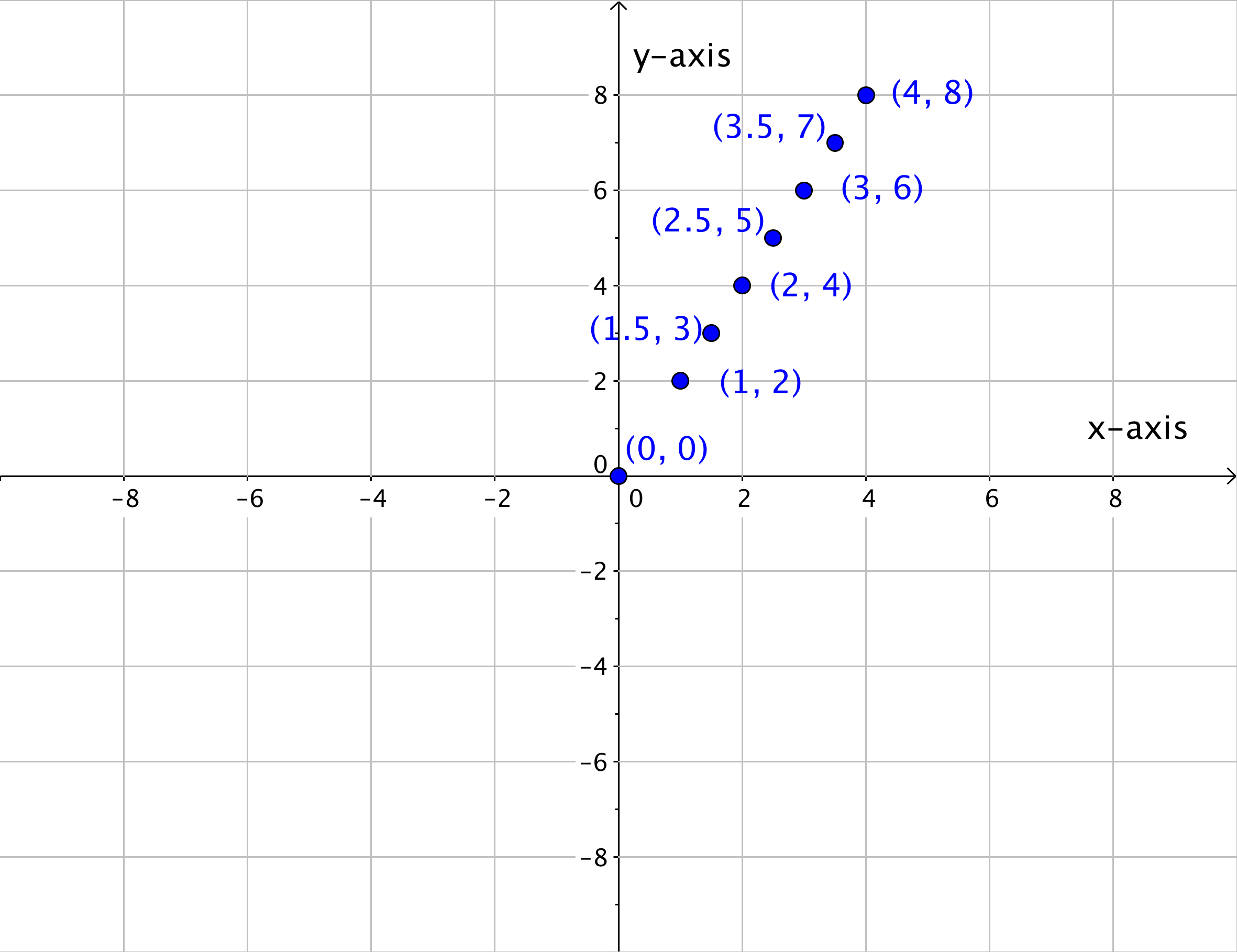Once you know the pattern that relates the x- and y-values, you can find a y-value for any x-value that lies on the line. So if the rule of this pattern is that each y-coordinate is twice the corresponding x-value, then the ordered pairs (1.5, 3), (2.5, 5), and (3.5, 7) should all appear on the line too, correct? Look to see what happens. Graph with the point (0,0); the point (1,2); the point (1.5, 3); the point (2,4); the point (2.5, 5); the point (3,6); the point (3.5, 7); and the point (4,8).

Graph with the point (0,0); the point (1,2); the point (1.5, 3); the point (2,4); the point (2.5, 5); the point (3,6); the point (3.5, 7); and the point (4,8).

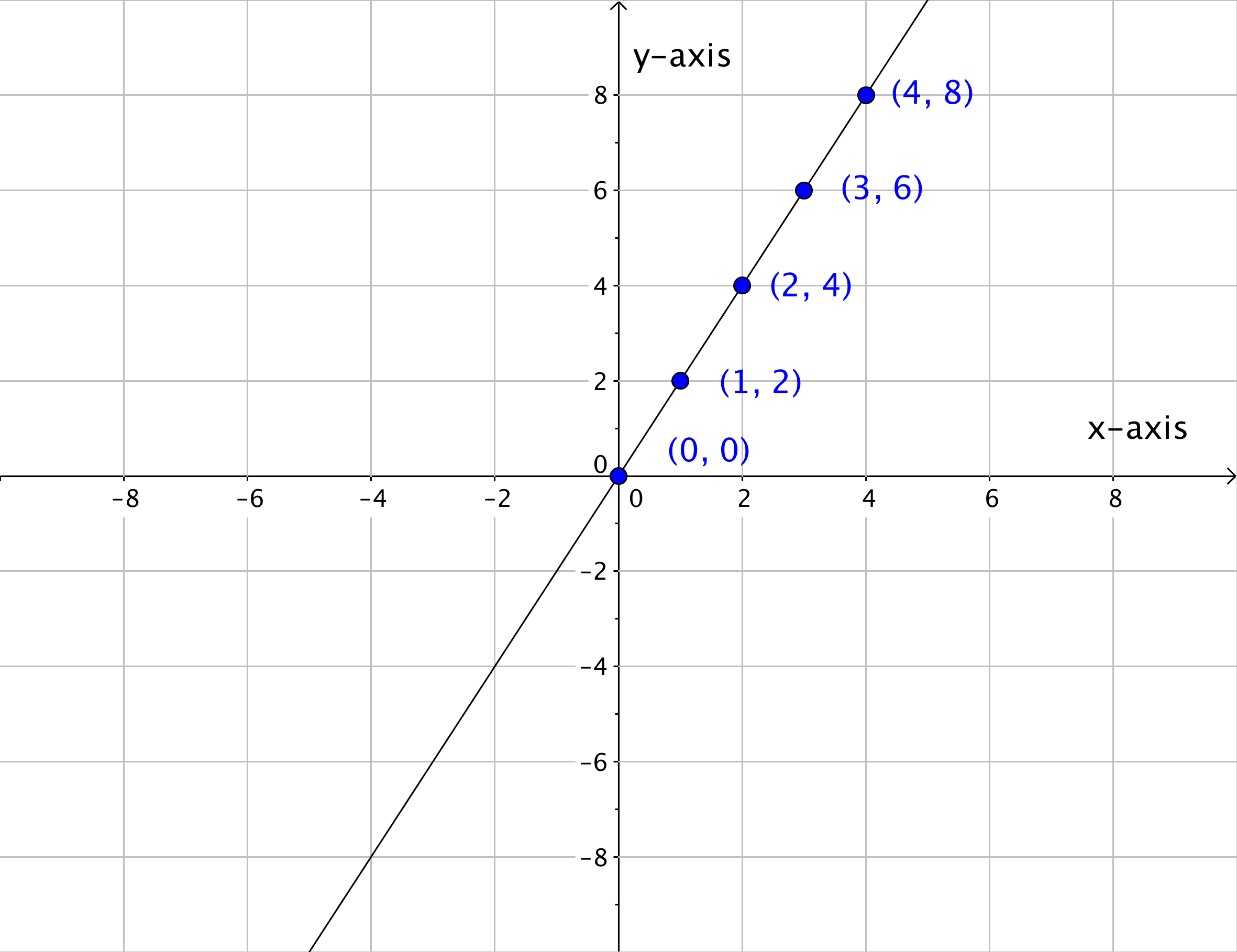If you were to keep adding ordered pairs (x, y) where the y-value was twice the x-value, you would end up with a graph like this. A line drawn through the point (0,0); the point (1,2); the point (2,4); the point (3,6); and the point (4,8).

A line drawn through the point (0,0); the point (1,2); the point (2,4); the point (3,6); and the point (4,8).

Look at how all of the points blend together to create a line. You can think of a line, then, as a collection of an infinite number of individual points that share the same mathematical relationship. In this case, the relationship is that the y-value is twice the x-value. There are multiple ways to represent a linear relationship—a table, a linear graph, and there is also a linear equation. A linear equation is an equation with two variables whose ordered pairs graph as a straight line. There are several ways to create a graph from a linear equation. One way is to create a table of values for x and y, and then plot these ordered pairs on the coordinate plane. Two points are enough to determine a line. However, it’s always a good idea to plot more than two points to avoid possible errors. Then you draw a line through the points to show all of the points that are on the line. The arrows at each end of the graph indicate that the line continues endlessly in both directions. Every point on this line is a solution to the linear equation.

Example

Graph the linear equation [latex]y=2x+3[/latex].

Answer: Evaluate [latex]y=2x+3[/latex] for different values of x, and create a table of corresponding x and y values.

x values	[latex]2x+3[/latex]	y values
0	2(0) + 3	3
1	2(1) + 3	5
2	2(2) + 3	7
3	2(3) + 3	9

(0, 3)

(1, 5)

(2, 7)

(3, 9)

Convert the table to ordered pairs. Plot the ordered pairs.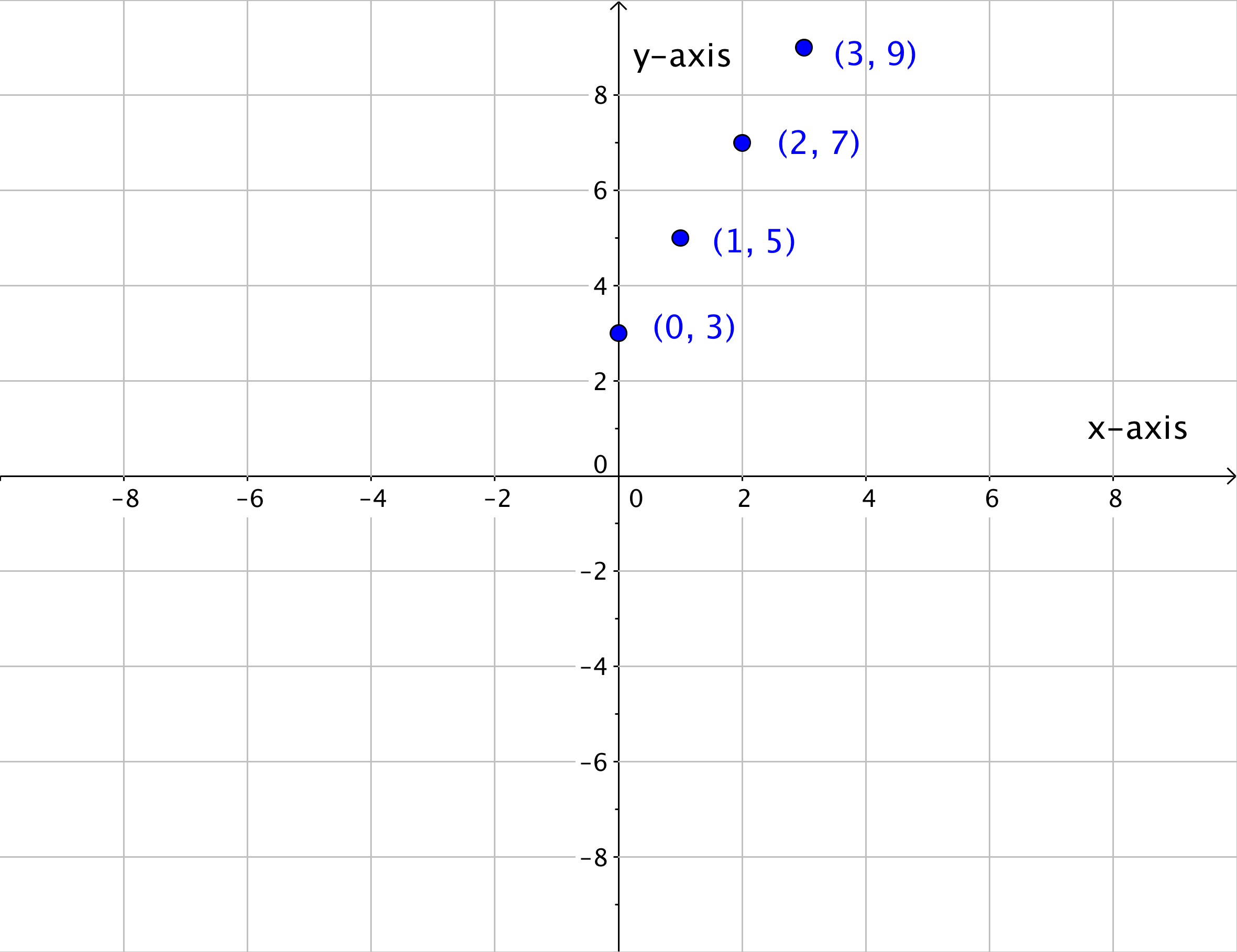 Graph showing the point (0,3); the point (1,5); the point (2,7); and the point (3,9).

Draw a line through the points to indicate all of the points on the line.

Answer

Line drawn through the point (0,3); the point (1,5); the point (2,7); and the point (3,9). The line is labeled y=2x+3.

Ordered Pairs as Solutions

So far, you have considered the following ideas about lines: a line is a visual representation of a linear equation, and the line itself is made up of an infinite number of points (or ordered pairs). The picture below shows the line of the linear equation [latex]y=2x–5[/latex] with some of the specific points on the line.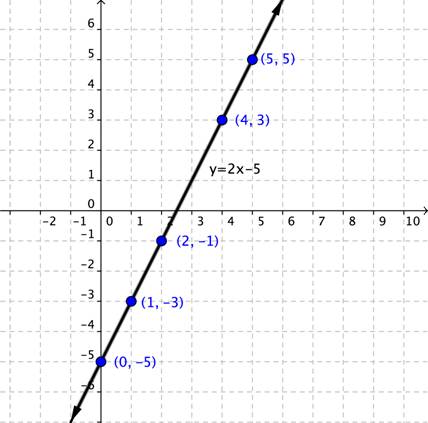 Line drawn through the points 0, negative 5; the point 1, negative 3; the point 2, negative 1; the point (4,3); and the point 5,5). The line is labeled y=2x-5.

Line drawn through the points 0, negative 5; the point 1, negative 3; the point 2, negative 1; the point (4,3); and the point 5,5). The line is labeled y=2x-5.

Every point on the line is a solution to the equation [latex]y=2x–5[/latex]. You can try any of the points that are labeled like the ordered pair, [latex](1,−3)[/latex].

[latex]\begin{array}{l}\,\,\,\,y=2x-5\\-3=2\left(1\right)-5\\-3=2-5\\-3=-3\\\text{This is true.}\end{array}[/latex]

You can also try ANY of the other points on the line. Every point on the line is a solution to the equation [latex]y=2x–5[/latex]. All this means is that determining whether an ordered pair is a solution of an equation is pretty straightforward. If the ordered pair is on the line created by the linear equation, then it is a solution to the equation. But if the ordered pair is not on the line—no matter how close it may look—then it is not a solution to the equation.

Identifying Solutions

To find out whether an ordered pair is a solution of a linear equation, you can do the following:

Graph the linear equation, and graph the ordered pair. If the ordered pair appears to be on the graph of a line, then it is a possible solution of the linear equation. If the ordered pair does not lie on the graph of a line, then it is not a solution.
Substitute the (x, y) values into the equation. If the equation yields a true statement, then the ordered pair is a solution of the linear equation. If the ordered pair does not yield a true statement then it is not a solution.

Example

Determine whether [latex](−2,4)[/latex] is a solution to the equation [latex]4y+5x=3[/latex].

Answer: For this problem, you will use the substitution method. Substitute [latex]x=−2[/latex] and [latex]y=4[/latex] into the equation.

[latex]\begin{array}{r}4y+5x=3\\4\left(4\right)+5\left(−2\right)=3\end{array}[/latex]

Evaluate.

[latex]\begin{array}{r}16+\left(−10\right)=3\\6=3\end{array}[/latex]

The statement is not true, so [latex](−2,4)[/latex] is not a solution to the equation [latex]4y+5x=3[/latex].

Answer

[latex](−2,4)[/latex] is not a solution to the equation [latex]4y+5x=3[/latex].

https://youtu.be/9aWGxt7OnB8

Intercepts

The intercepts of a line are the points where the line intercepts, or crosses, the horizontal and vertical axes. To help you remember what “intercept” means, think about the word “intersect.” The two words sound alike and in this case mean the same thing. The straight line on the graph below intercepts the two coordinate axes. The point where the line crosses the x-axis is called the x-intercept. The y-intercept is the point where the line crosses the y-axis.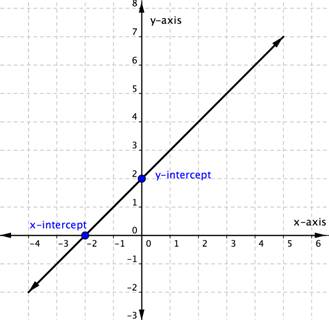 A line going through two points. One point is on the x-axis and is labeled the x-intercept. The other point is on the y-axis and is labeled y-intercept.

A line going through two points. One point is on the x-axis and is labeled the x-intercept. The other point is on the y-axis and is labeled y-intercept.

The x-intercept above is the point [latex](−2,0)[/latex]. The y-intercept above is the point (0, 2). Notice that the y-intercept always occurs where [latex]x=0[/latex], and the x-intercept always occurs where [latex]y=0[/latex]. To find the x- and y-intercepts of a linear equation, you can substitute 0 for y and for x respectively. For example, the linear equation [latex]3y+2x=6[/latex] has an x intercept when [latex]y=0[/latex], so [latex]3\left(0\right)+2x=6\\[/latex].

[latex]\begin{array}{r}2x=6\\x=3\end{array}[/latex]

The x-intercept is [latex](3,0)[/latex]. Likewise the y-intercept occurs when [latex]x=0[/latex].

[latex]\begin{array}{r}3y+2\left(0\right)=6\\3y=6\\y=2\end{array}[/latex]

The y-intercept is [latex](0,2)[/latex].

Using Intercepts to Graph Lines

You can use intercepts to graph linear equations. Once you have found the two intercepts, draw a line through them. Let’s do it with the equation [latex]3y+2x=6[/latex]. You figured out that the intercepts of the line this equation represents are [latex](0,2)[/latex] and [latex](3,0)[/latex]. That’s all you need to know.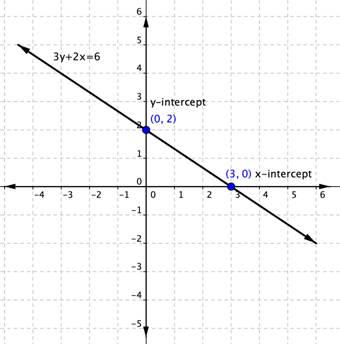 A line drawn through the points (0,2) and (3,0). The point (0,2) is labeled y-intercept and the point (3,0) is labeled x-intercept. The line is labeled 3y+2x=6.

A line drawn through the points (0,2) and (3,0). The point (0,2) is labeled y-intercept and the point (3,0) is labeled x-intercept. The line is labeled 3y+2x=6.

Example

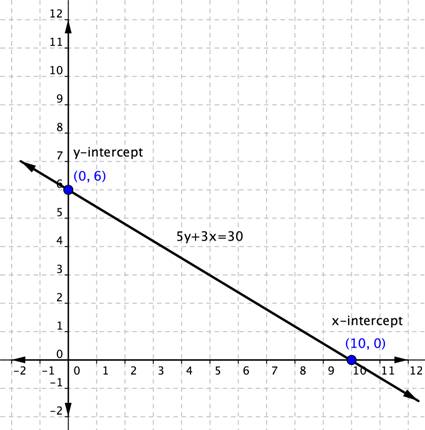
Graph [latex]5y+3x=30[/latex] using the x and y-intercepts.

Answer: When an equation is in [latex]Ax+By=C[/latex] form, you can easily find the x- and y-intercepts and then graph.

[latex]\begin{array}{r}5y+3x=30\\5y+3\left(0\right)=30\\5y+0=30\\5y=30\\y=\,\,\,6\\y\text{-intercept}\,\left(0,6\right)\end{array}[/latex]

To find the y-intercept, set [latex]x=0[/latex] and solve for y.

[latex]\begin{array}{r}5y+3x=30\\5\left(0\right)+3x=30\\0+3x=30\\3x=30\\x=10\\x\text{-intercept}\left(10,0\right)\end{array}[/latex]

To find the x-intercept, set [latex]y=0[/latex] and solve for x.

Answer

https://youtu.be/k8r-q_T6UFk

Example

Graph [latex]y=2x-4[/latex] using the x and y-intercepts.

Answer: First, find the y-intercept. Set x equal to zero and solve for y.

[latex]\begin{array}{l}y=2x-4\\y=2\left(0\right)-4\\y=0-4\\y=-4\\y\text{-intercept}\left(0,-4\right)\end{array}[/latex]

To find the x-intercept, set [latex]y=0[/latex] and solve for x.

[latex]\begin{array}{l}y=2x-4\\0=2x-4\\4=2x\\x=2\\x\text{-intercept}\left(2,0\right)\end{array}[/latex]

Answer

Graphing other equations using a table of values

Example

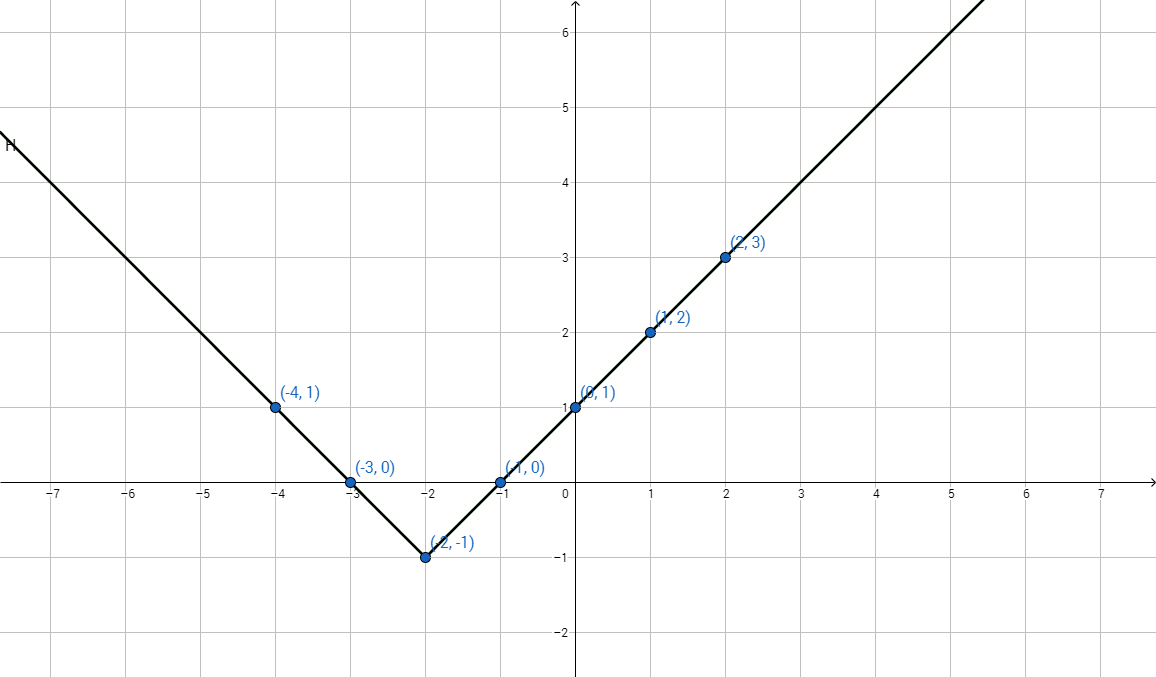
Graph the equation [latex]y=|x+2|-1[/latex].

Answer: Evaluate [latex]y=|x+2|-1[/latex] for different values of x, and create a table of corresponding x and y values.

x values	[latex]\|x+2\|-1[/latex]	y values
-4	[latex] \|(-4)+2\|-1[/latex]	1
-3	[latex] \|(-3)+2\|-1[/latex]	0
-2	[latex] \|(-2)+2\|-1[/latex]	-1
-1	[latex] \|(-1)+2\|-1[/latex]	0
0	[latex] \|(0)+2\|-1[/latex]	1
1	[latex]\|(1)+2\|-1[/latex]	2
2	[latex] \|(2)+2\|-1[/latex]	3

(-4,1)

(-3, 0)

(-2,-1)

(-1, 0)

(0, 1)

(1, 2)

(2, 3)

Convert the table to ordered pairs. Plot the ordered pairs.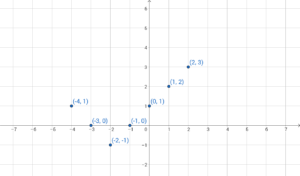

Draw a line through the points to indicate all of the points on the line.

Answer

Try It

[ohm_question]63436[/ohm_question]

Licenses & Attributions

CC licensed content, Original

Authored by: Quadrants on the Coordinate Plane. License: CC BY: Attribution.

CC licensed content, Shared previously

Graph Basic Linear Equations by Completing a Table of Values. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
Determine If an Ordered Pair is a Solution to a Linear Equation. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
Plot Points Given as Ordered Pairs on the Coordinate Plane. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.

x values	[latex]\|x+2\|-1[/latex]	y values
-4	[latex] \|(-4)+2\|-1[/latex]	1
-3	[latex] \|(-3)+2\|-1[/latex]	0
-2	[latex] \|(-2)+2\|-1[/latex]	-1
-1	[latex] \|(-1)+2\|-1[/latex]	0
0	[latex] \|(0)+2\|-1[/latex]	1
1	[latex]\|(1)+2\|-1[/latex]	2
2	[latex] \|(2)+2\|-1[/latex]	3

Study Guides > College Algebra: Co-requisite Course

Coordinate Plane and Graphing Equations

Learning Objectives

Example

Answer

Example

Plotting points to graph linear relationships

Example

Answer

Ordered Pairs as Solutions

Identifying Solutions

Example

Answer

Intercepts

Using Intercepts to Graph Lines

Example

Answer

Example

Answer

Graphing other equations using a table of values

Example

Answer

Try It

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously