Section Exercises
1. How can you use factoring to simplify rational expressions? 2. How do you use the LCD to combine two rational expressions? 3. Tell whether the following statement is true or false and explain why: You only need to find the LCD when adding or subtracting rational expressions. For the following exercises, simplify the rational expressions. 4. 5. 6. 7. 8. 9. 10. 11. 12. 13. For the following exercises, multiply the rational expressions and express the product in simplest form. 14. 15. 16. 17. 18. 19. 20. 21. 22. 23. For the following exercises, divide the rational expressions. 24. 25. 26. 27. 28. 29. 30. 31. 32. For the following exercises, add and subtract the rational expressions, and then simplify. 33. 34. 35. 36. 37. 38. 39. 40. 41. For the following exercises, simplify the rational expression. 42. 43. 44. 45. 46. 47. 48. 49. 50. 51. Brenda is placing tile on her bathroom floor. The area of the floor is ft2. The area of one tile is . To find the number of tiles needed, simplify the rational expression: .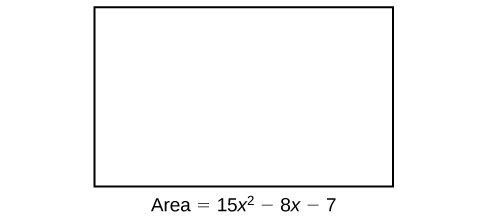 52. The area of Sandy’s yard is ft2. A patch of sod has an area of ft2. Divide the two areas and simplify to find how many pieces of sod Sandy needs to cover her yard.
53. Aaron wants to mulch his garden. His garden is ft2. One bag of mulch covers ft2. Divide the expressions and simplify to find how many bags of mulch Aaron needs to mulch his garden.
For the following exercises, perform the given operations and simplify.
54.
55.
56.
57.
52. The area of Sandy’s yard is ft2. A patch of sod has an area of ft2. Divide the two areas and simplify to find how many pieces of sod Sandy needs to cover her yard.
53. Aaron wants to mulch his garden. His garden is ft2. One bag of mulch covers ft2. Divide the expressions and simplify to find how many bags of mulch Aaron needs to mulch his garden.
For the following exercises, perform the given operations and simplify.
54.
55.
56.
57.
